Filling the cube
Tomoko Fuse has written (drawn) a book " "
(ISBN 4-480-87263-9).
Some people say, that this title means "Fun filled boxes".
You can find in this book partition of a cube into pieces.
These pieces are divided into smaller pieces subdivided into more small pieces.
See below for some examples of such partition.
"
(ISBN 4-480-87263-9).
Some people say, that this title means "Fun filled boxes".
You can find in this book partition of a cube into pieces.
These pieces are divided into smaller pieces subdivided into more small pieces.
See below for some examples of such partition.
Join every vertex of a cube with the center of the cube and divide a cube into 6 congruent pyramids.
Puzzle: what happens if you stick these pyramids to the cube faces outside ?
You can divide every pyramid into 2 smaller parts (along the diagonal of the base or parallel to the side of the base),
into 4 smaller parts (along the both diagonals or parallel to the both sides of the base),
into 8 smallest parts (along the both diagonals and parallel to the both sides of the base),
This way you can divide a cube into 12, 24 or 48 equal parts.
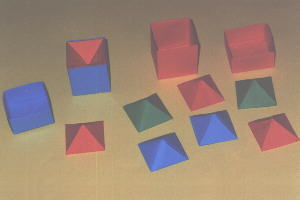
The following photo shows division into 48 parts
(one large pyramid is decomposed, other 5 are arranged in the box).
This cube is divided into small, smaller and smallest parts of different shape.

It's a lot of fun when you pull parts out the cube and much more when you put parts back.
Be careful with spare parts remaining outside box when you fill it completely :-)
In the second book " "
(ISBN 4-416-38823-3)
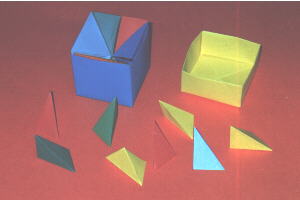
Tomoko Fuse "rams" solids into other solids.
The following photos shows a tetrahedron put into a cube.
"
(ISBN 4-416-38823-3)
Tomoko Fuse "rams" solids into other solids.
The following photos shows a tetrahedron put into a cube.


© Copyright K. & W. Burczyk, 1999
Last modified:
 "
(ISBN 4-480-87263-9).
Some people say, that this title means "Fun filled boxes".
You can find in this book partition of a cube into pieces.
These pieces are divided into smaller pieces subdivided into more small pieces.
See below for some examples of such partition.
"
(ISBN 4-480-87263-9).
Some people say, that this title means "Fun filled boxes".
You can find in this book partition of a cube into pieces.
These pieces are divided into smaller pieces subdivided into more small pieces.
See below for some examples of such partition.



 "
(ISBN 4-416-38823-3)
Tomoko Fuse "rams" solids into other solids.
The following photos shows a tetrahedron put into a cube.
"
(ISBN 4-416-38823-3)
Tomoko Fuse "rams" solids into other solids.
The following photos shows a tetrahedron put into a cube.

